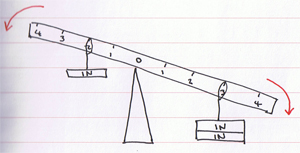
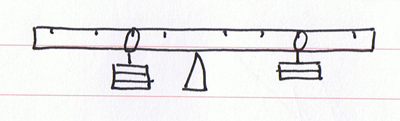
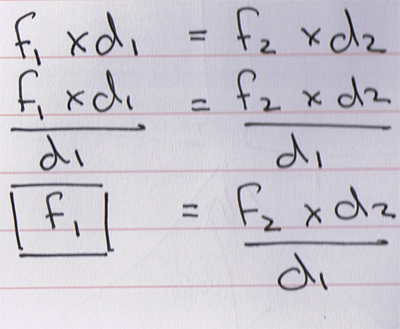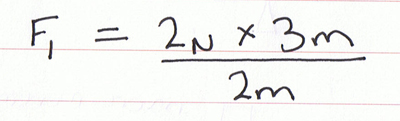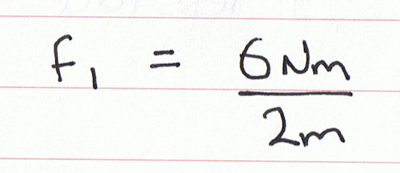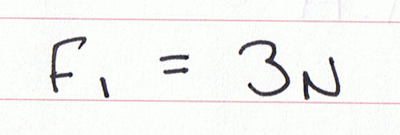Turning motions are really easy to understand, take this set of scales:

The right hand side is turnign clockwise and the left hand side is turning anti clockwise.

To balance the scales or bring them into equilibrium the clockwise turning motion has to balance the anticlockwise motion. To work this out you need to use a famous equation:

Force x Distance = Moment 

A moment is a turning force and is measured in Newton meters or Nm.

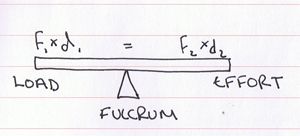
You can turn our set of scales into a lever of the first order to better understand this equation:

You have to do the equation for both sides to get them to balance so in this instance our unknown is the load on the left hand side of our scale so our equation would look like this:

 F1 x 2m = 2N x 3m

By breaking this down into two separate equations we can work this out:

2N x 3m = 6Nm

 F1 x 2m = 6Nm

You have to do something very mathsy now called transposing formula, basically moving the elements of the formula around to get F1 in the right place. As long as you do the same to both sides of the equation it remains true, here’s some examples:

3 + 4 = 7

2 + 3 + 4 = 7 + 2

√3 + 4 = √7

You also need to remember this rule : is you change the side you change the sign! For example:

3 x 4 = 12

3 = 12 ÷4

So our moments formula needs to undergo a bit of a transformation before we can use it:

So we end up with:

By using this result we now know that to bring out imbalanced set of scales into equilibrium we need to add an extra 2 N to the left hand side!